 |





|
Consecutive Fifths & Octaves in the Bach Chorales
Avoiding parallel fifths and octaves is one of the foundational "rules" of counterpoint. While no thorough account of the rationale behind this rule will be rehearsed here, suffice it to say that parallel fifths and octaves violate the principles of counterpoint by interfering with the independence of voices required. While this rule refers to parallel fifths/octaves — that is, consecutive harmonic perfect fifths and octaves in parallel contrapuntal motion — consecutive fifths or octaves in contrary motion are categorically the same and are also to be avoided. (In other words, parallel fifths/octaves cannot be corrected through octave transference.) Because of this, the forbidden fifths/octaves under discussion here will be referred to as "consecutive fifths/octaves" or even more simply "consecutives."
Do consecutives fifths and octaves occur in Bach’s chorales?
Yes, they do. In fact, no fewer than 54 instances of consecutives perfect fifths and octaves can be found in the chorales. But exactly what kind of consecutives did Bach write and in what specific contexts? Did he write consecutive octaves or fifths only? Did he only write parallels that involve non–chord tones (i.e. parallels that disappear with the removal of NCTs)? To help give a clearer picture regarding questions of these types, the 54 instances of consecutives are categorized below.
"Fermata" Consecutives
Over half of the 54 consecutives occur between the final chord of one phrase and the first chord of the next. These "fermata" consecutives can to a significant degree be considered non–syntactical since they occur between two separate phrases. Any negative contrapuntal effect of these consecutives is significantly attenuated if not eliminated entirely.
Here are 29 "fermata consecutives" among the chorales:
| BWV | Riemens. | Measure | Voices | Description |
|---|---|---|---|---|
| 60.5 | 216 | 6 | S/B | consecutive P8s in contrary motion |
| 78.7 | 297 | 4–5 | S/B | consecutive P5s in contrary motion |
| 92.9 | X | 14 | S/B | consecutive P5s in contrary motion |
| 99.6 | X | 12 | A/B | consecutive P5s in contrary motion |
| 108.6 | 45 | 4 | T/B | consecutive P5s in contrary motion |
| 111.6 | X | 15 | S/B | consecutive P5s in contrary motion |
| 115.6 | 38 | 10–11 | A/T | consecutive P5s in contrary motion |
| 157.5 | X | 9–10 | A/B | consecutive P5s in contrary motion |
| 174.5 | 58 | 23 | A/T | consecutive P5s in parallel motion |
| 183.5 | 123 | 14 | S/T | consecutive P5s in contrary motion |
| 190.7 | 327 | 18 | A/B | consecutive P5s in parallel motion |
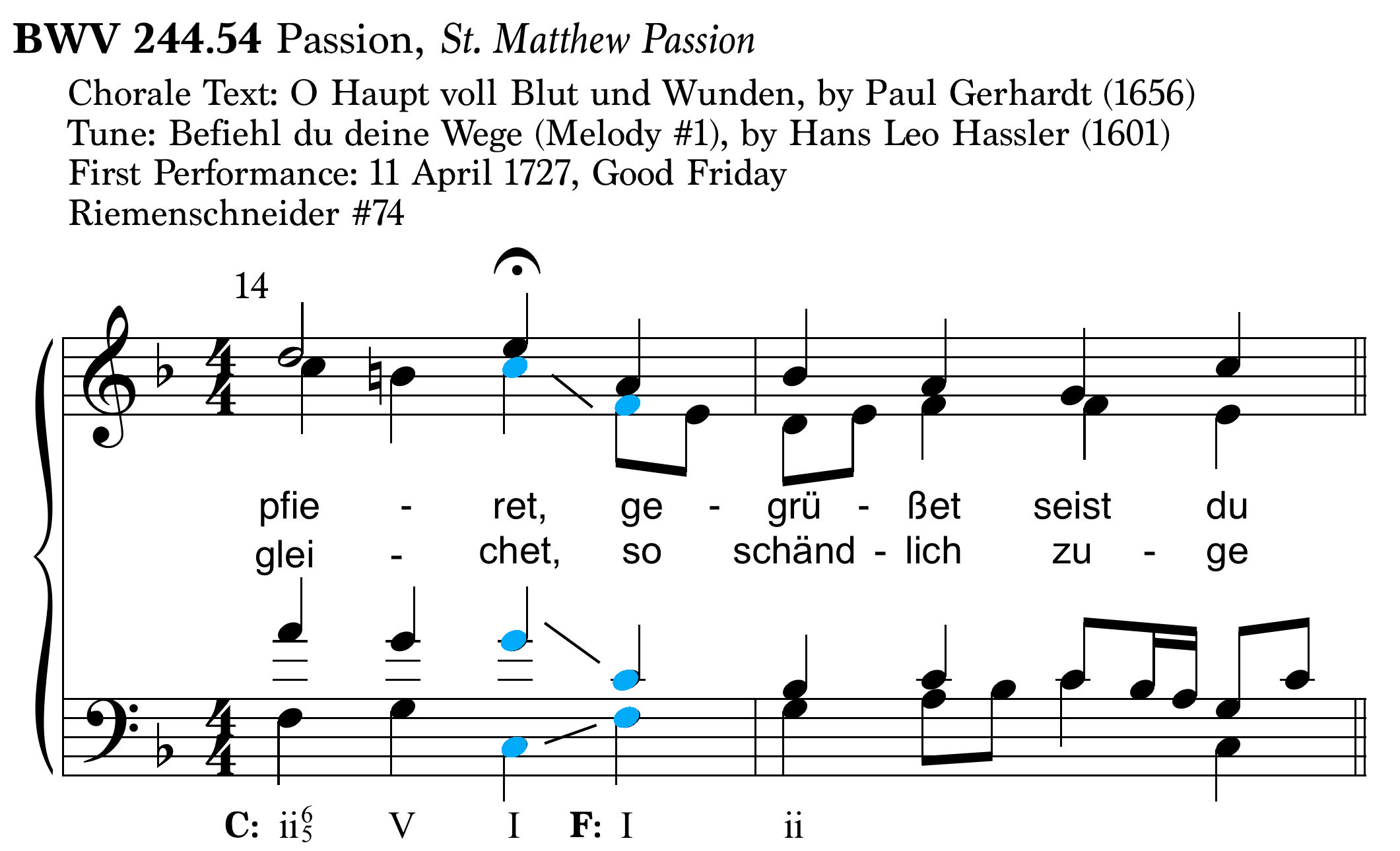
| 244.44 | 80 | 14 | A/T/B | consecutive P5s (T/B) & P8s (A/B) in contrary motion |
| 244.54 | 74 | 14 | A/T/B | consecutive P5s (T/B) & P8s (A/B) in contrary motion |
| 244.62 | 89 | 14 | A/T/B | consecutive P5s (T/B) & P8s (A/B) in contrary motion |
| 245.40 | 107 | 23 | S/T | consecutive P5s in contrary motion |
| 266 | 208 | 5 | A/B | consecutive P5s in contrary motion |
| 301 | 134 | 3 | T/B | consecutive P5s in parallel motion |
| 326 | 164 | 8 | A/B | consecutive P8s (A/B) in parallel motion |
| 329 | 212 | 5 | S/T/B | consecutive P5s (T/B) & P8s (S/B) in contrary motion |
| 333 | 226 | 12 | A/T/B | P5s in contrary motion (T/B) & P8s in parallel motion (A/B) |
| 340 | 277 | 21 | S/B | consecutive P5s in contrary motion |
| 347 | 2 | 14 | A/B | consecutive P8s in contrary motion |
| 385 | 36 | 6 | T/B | parallel P5s (melodically up a P8) |
| 436 | 278 | 18 | A/B | consecutive P5s in contrary motion |
Non–Structural Consecutives
Setting aside these 29 non–syntactical consecutives, 25 mid–phrase consecutives remain. Of those 25, fourteen of them constitute consecutive involving non–chord tones (NCTs) such as passing tones, neighbor tones, anticipations, etc. Remove these embellishing notes and the objectionable consecutive P5 or P8 disappears. Such "non–structural" consecutives may be considered less objectionable than structural consecutives but are generally still regarding as problematic (all contextual factors being equal). Of these fourteen non–structural parallels, nine represent a very specific type of parallel fifths referred to here as "cadential parallels" in which a Re–Do anticipation figure in the soprano coincides with a Sol–Fa delayed arrival of the seventh in a V7 chord to create parallel fifths.

Here are the fourteen "non–structural" consecutives found in the Bach chorales:
| BWV | Riemens. | Measure | Voices | Description |
|---|---|---|---|---|
| 26.6 | 48 | 4 | S/T | "cadential" parallel fifths |
| 33.6 | 13 | 2–3 | S/T | m.2 beat 4 to m.3 beat 1: parallel P5s |
| 40.8 | 8 | 2 | S/T | "cadential" parallel fifths |
| 40.8 | 8 | 4 | S/T | "cadential" parallel fifths |
| 40.8 | 8 | 6 | S/T | "cadential" parallel fifths |
| 40.8 | 8 | 16 | S/T | "cadential" parallel fifths |
| 48.7 | 266 | 14 | A/T | beats 1–2: parallel P5s, passing tone in A. |
| 99.6 | X | 11 | S/T | beats 3–4: parallel P5s, neighbor tone in S. |
| 146.8 | X | 10 | S/T | "cadential" parallel fifths |
| 167.5 | X | 11/25–12/26 | T/B | parallel P8s! Incomplete neighbor in T. |
| 244.40 | 121 | 4 | S/T | "cadential" parallel fifths |
| 248.33 | 139 | 2 | S/A | beats 1–2: parallel P5s, passing tone in S. |
| 263 | 128 | 6 | S/T | "cadential" parallel fifths |
| 361 | 264 | 12 | S/T | "cadential" parallel fifths |
Structural Consecutives
The remaining eleven instances of consecutives are legitimate structural, chordal, syntactical, mid–phrase consecutives that fall directly within in the paradigm of objectionable parallels, though contextual factors in each case certainly attenuate the negative aural effects of such problematic contrapuntal devices.
| BWV | Riemens. | Measure | Voices | Description |
|---|---|---|---|---|
| 7.7 | X | 2/6 | T/B | beat 1: consecutive P5s |
| 22.5 | X | 27–28 | T/B | m.27 beat 4 to m.28 beat 1: P8s in contrary motion |
| 33.6 | 13 | 14 | S/T | beats 2–3: P5s in contrary motion |
| 86.6 | 4 | 13–14 | S/T | m.13 beat 4 to m.14 beat 1: parallel P5s |
| 245.40 | 107 | 23 | T/B | beats 3–4: P8s in contrary motion |
| 251 | 329 | 13–14 | S/T | m.13 beat 4 to m.14 beat 1: P8s in contrary motion |
| 278 | 371 | 3/7 | S/T | beats 2–3: parallel P8s in parallel motion |
| 306 | 176 | 13–14 | S/T | m.13 beat 3 to m.14 beat 1: parallel P8s |
| 308 | 27 | 9 | T/B | beats 3–4: P5s in contrary motion |
| 323 | 320 | 8 | S/T | beats 1–3: parallel P5s |
| 393 | 275 | 5 | A/T | beats 3–4: P5s in contrary motion |
Conclusion
While it may be a bit surprising to find so many instances of consecutives among the chorales of Bach, it is worth remembering that given the large number of chorales (more than 400), 25 instances of syntactical consecutives is an extremely small number — a ratio of approximately one instance per 224 measures of four–part counterpoint. Eliminating the nine "cadential" parallels as a particular type of consecutives that Bach notably allowed, the remaining fourteen instances of consecutives equate to about one instance per 350 measures!
The question of why Bach wrote these objectionable consecutives that he did, particularly the structural ones, many of which are quite fixable, is difficult to answer. Malcolm Boyd has suggested that Bach, who often quickly tossed off his cantata–ending chorales at the end of the week prior to Sunday services in Leipzig, simply overlooked these consecutives. They are truly mistakes. Others have challenged this idea, suggesting that Bach, the consumate musician who was never bound by "rules" in the first place, did not consider such consecutives to be objectionable. In reality, both explanations arrive at nearly the same conclusion — if these consecutives truly are mistakes, then they are mistakes that Bach’s musical ear considered allowable.
One important followup question remains: Based on the above data presented here, just what kinds of syntactical consecutives did Bach (or his ear) consider allowable? A thorough answer will not be attempted here, but some general conclusions can be made:
1) Bach did not consider "cadential parallels" to be objectionable, given the number of times they appear.
2) Consecutive fifths are less objectionable than octaves.
3) Consecutives in contrary motion are less objectionable than in parallel motion.
4) Bach’s syntactical consecutives always involves an inner voice — no consecutives between soprano and bass can be found!
5) Only a single instance (BWV 306) of stepwise, chordal consecutives in parallel motion occurs, in more than 5600 measures of part–writing!
One Final Note: Editorial "Corrections"
The data above shows a disproportionate percentage of consecutives coming from BWV 1–252 which represents works for which original manuscripts are extant. (The individual BWV 253–438 chorales, on the other hand, have survived by way of secondary sources, like the Breitkopf edition first published in the 1780s.) There is a logical reason for this discrepancy. When comparing BWV 1–252 chorales as they appear in the original manuscripts against those same settings as presented in early collections, we find that the editors of these early collections took liberties in "correcting" many of the consecutives listed above. Of the six "cadential parallels", all six are "corrected" in the Breitkopf edition, generally by staggering the parallels rhythmically (delaying the soprano or tenor by a sixteenth note). In the Fasch manuscript that predates the Breitkopf, only four of the six cadential parallels are corrected. And in the manuscript AmB 46IIa, four of six are corrected, though not the same four as the Fasch corrects and the corrections themselves are not consistent. (For example, in BWV 40.8 measure 4, the Breitkopf and Fasch delay the soprano by a sixteenth, while in AmB 46IIa it is the tenor that is delayed one sixteenth.)
Of the two other "non–structural" consecutives from BWV 1–252 that appear in the Breitkopf (BWVs 48.7 and 248.33), both are corrected in the Breitkopf. Interestingly, however, the three "structural" consecutives that appear in the Breitkopf are left uncorrected. This curious fact would seem to suggest that these editors considered consecutives involving non–chord tones to be more objectionable that chordal consecutives, though the sample size is certainly small.
Naturally, since the BWV 253–438 have survived only by way of these early chorale collections, we have no way of knowing where editorial corrections have been exacted since we have no original manuscripts against which to compare these settings. We can, however, compare the 48 chorales from BWV 253–438 that appear in the early Dietel collection from the mid–1730s against that source, since Ludwig Dietel almost certainly copied his manuscript directly from Bach’s original manuscripts in Leipzig. In doing so, we find six additional consecutives (two cadential parallels, two other non–structural consecutives, and two structural consecutives) in the Dietel that are corrected in the Breitkopf, raising the overall total of consecutives among the chorales from 50 to 56. Accounting for these additional instances, the number of consecutives per measure among these 48 Dietel settings arrives at a very similar ratio to that represented in the BWV 1–252 settings from original sources. (One difficulty in the enterprise of comparing Breitkopf chorales against the Dietel is that Dietel was not terribly accurate as a copyist. Still, given the early date of the manuscript, it is a worthwhile endeavor.)
The six additional Dietel consecutives:
| Dietel | BWV | Riemen. | Measure | Voices | Description |
|---|---|---|---|---|---|
| 7 | 362 | 252 | 5/13 | A/T | beat 2: in Dietel, A. has upper neighbor (A–Bb–A) rather than lower neighbor (A–G–A), creating parallel fifths w/ T. (Eb–D) |
| 23 | 397 | 274 | 18–19 | S/T | m.18, beat 4 to m.19, beat 1: cadential parallels. In Dietel, T. has straight eighths (D–C) |
| 57 | 393 | 275 | 5 | S/T | beats 1–2: parallel octaves. Dietel has A–B quarters rather than C#–B. |
| 57 | 393 | 275 | 12 | S/B | beat 1: Dietel has C#–F# eighths rather than E–D#, creating parallel P5s w/ S. |
| 104 | 360 | 350 | 12 | S/T | beats 2–3: cadential parallels. Dietel has straight eighths in S. (F–Eb) |
| 109 | 385 | 36 | 6 | B/T | beat 4: Dietel has C#4–E#3 eighths in B. rather than F#–E#, creating poorly masked parallel fifths w/ T. from beat 3 to 4. |
bach–chorales.com by Luke Dahn. Copyright 2018.